An SCA survey on the calculations of drainage capillary pressure curves from centrifuge measurements
Introduction
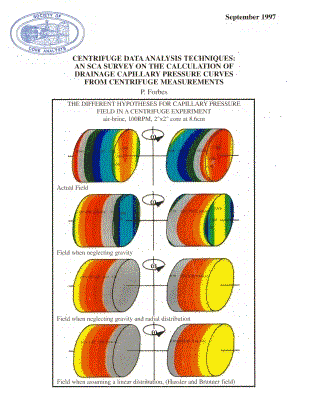
In 1993 the Society of Core Analysts, SCA, completed an inter-laboratory survey on drainage capillary pressure measurement by centrifuge. The aim of the study was to evaluate how the different methods of implementing the centrifuge technique impact the results.
Companion 1″ x l” samples of Berea sandstone and Bedford Limestone were characterised, in term of porosity, grain density and permeability, and mailed world-wide to 24 laboratories for capillary pressure determination. Fifteen laboratories contributed, providing air displacing brine capillary pressure by centrifuge. Contributors included oil & gas companies, contractors and R&D institutes. Operating procedures were not specified but every laboratory was asked to provide a full description of processes and intermediate results. This was the first SCA survey on capillary pressure.
The results of that survey were analyzed and discussed during a workshop on August 9, 1993, in Houston (SCA, 1993, Ruth and Chen, 1995).
Significant differences between capillary pressure curves were found (Figure 1), even though the companion samples were comparable in term of porosity and permeability. The analysis focused on ways to improve and eventually standardise experimental design, measurements, and interpretation.
Many fundamental problems, concerning flow mechanisms in a centrifuge experiment, were addressed and recommendations were provided for designing centrifuge experiments. However, it was impossible to isolate the source of the variability, whether this was from measurements or from interpretation processes.
Indeed, in the centrifuge method, the capillary pressure curve is not directly measured, but is calculated, from fluid production measurements, using various approximate methods. During the SCA 1993 workshop, these methods were also discussed (Forbes, 1993). It appeared that the choice of the calculation procedure may potentially affect the resultant capillary pressure curve. Accordingly, it
was decided to launch a second survey specific to the evaluation of the calculation methods for drainage centrifuge capillary pressure curves. That calculation survey is presented below.
Acknowledgements
The survey was performed thanks to the active participation of laboratories from different oil & gas companies, contractors or R&D institutes. Many thanks are expressed to all of them for their contribution. Jill Buckley and Andre Bouchard are thanked for their helpful comments and suggestions.
Highlights
(conclusions)
The present survey demonstrated that:
- The main source of inaccuracy in the drainage capillary pressure curve determination by centrifuge is related to the interpretation process and not to experimental procedures (assuming accepted procedures are in use) nor to shape of the capillary pressure curve.
- The inaccuracy depends on the centrifuge geometry (and resulting contributions of centrifugal and radial effects) and on the method used for solving the centrifuge equation. The choice of the method, according to the centrifuge/core geometry, appears to be of prime importance, much more than improving experimental conditions. This is especially critical for short radius geometry, and includes the most common geometry of 1″ x l” sample run at 8.6 cm from the centrifuge axis.
- Additional errors due to the inversion process may be very large (+/-10 saturation units). They cannot be reduced significantly by increasing the number of rotation steps or by reducing the experimental error below 2 saturation units or by averaging the results from different interpretation processes. Because most of these processes introduce a negative bias, the common practice of using different interpretations and averaging the results is totally irrelevant. Errors being dominantly negative, no compensation is achieved by averaging.
- The only way to improve the capillary pressure curve determination is to use an appropriate solution of the centrifuge equation, consistent with the centrifuge and core geometry.
- Whatever the geometry in use, only a few solutions may insure a reasonable accuracy, i.e. below +/- 3 saturation units. These are SPL (Nordtvedt et al., 1990) and S (Forbes, 1991).
- These solutions require a proper implementation and the use of correction for radial effects and (or) the use of consistency constraints.
- A figure is provided to evaluate the additional errors due to the interpretation process for most of the other solutions in use in the industry. Their accuracy is always improved by using radial correction or consistency constraints.



